Defining stranded or Litz wire inductors

Many different coils and inductors are used in induction heating process, one of them being stranded coil. Stranded coil refers to a coil consisting of many fine turns of conducting wires.

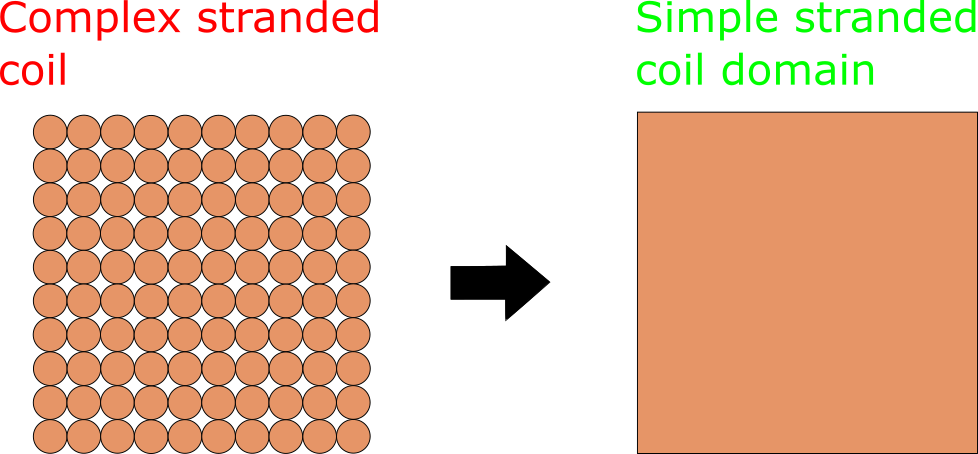
Electromagnetic analysis of a stranded coil can be complicated because the geometry of the coil is complex. CENOS gives you the advantage of defining a solid coil as stranded through a specific domain type, and ease the simulation of it to a minimum. CENOS allows you to define and simulate a specific stranded coil type, called Litz wire.

 

Difference Between Litz Wire and Non-Litz Wire

Litz wire consists of multiple strands of insulated wire twisted or braided together and connected in parallel, typically at the ends of the coil. In contrast, non-Litz wire is a single, continuous strand of wire.

When comparing the two with equivalent inputs:

• Non-Litz Wire:
  • Higher resistance.
  • Higher voltage.
  • This is due to the single strand carrying the entire current, increasing the resistive losses.
• Litz Wire:
  • Lower resistance.
  • Lower voltage.
  • This is because the current is distributed across multiple strands, reducing resistive losses due to their parallel connection.

When to use Stranded Coil domain?​

The Stranded Coil Source domain type is suitable for both 2D and 3D simulations of stranded coils, especially when the number of strands is too high to efficiently resolve them through explicit geometry.

By using the Stranded Coil domain type, you can:

• Reduce mesh element count and shorten simulation time, and
• Simplify geometry creation by modeling the coil as a solid body and defining it as stranded, without needing to manually create individual strands.

Important: To simulate a stranded coil, replace the coil geometry with an equivalent cross-sectional shape, define it as a Stranded Coil Source, and proceed with the calculation!

How to use Stranded Coil?​

You can easily access Stranded Coil Source domain under Domain type dropdown menu.

When the Stranded Coil Source is selected, the Domain Properties section will appear, containing four parameters:

• Number of Turns
• Fill Factor
• Cross-Sectional Area (specific to 3D definitions only).

The parameters Number of Turns, Fill Factor, and Cross-Sectional Area are specific to stranded coils and define the geometric distribution of strands within the solid inductor. Read more about them below.

To simulate a Litz wire inductor, simply enable the Litz wire checkbox.

Current​

The definition of Current depends on the type of stranded coil being used:

• Stranded Current Source: When using this domain type, the defined current represents the current in a single strand, as it assumes a single long strand forming all the loops.
• Litz Wire: If the Litz Wire checkbox is enabled, the total current is evenly distributed among all strands, as they are connected at both the beginning and end of the wire.

Examples:

• Switching to Litz wire:
  If you are transitioning from a solid inductor carrying 1000 A to a Litz wire with 10 strands and a total current of 1000 A, you should enter 1000 A in the Current (Amplitude) field.
• Switching to a simple Stranded Coil:
  If transitioning from a solid inductor carrying 1000 A to a simple stranded coil with 10 strands and a total current of 1000 A, you need to enter 100 A in the Current (Amplitude) field. This ensures the same current density across the wire’s cross-sectional area.

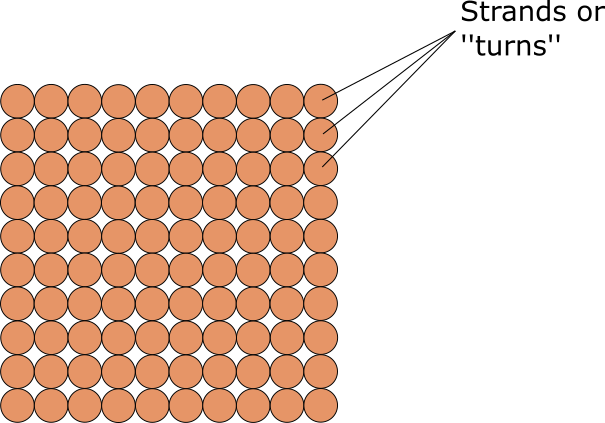
Number of turns​

Each strand of a stranded coil is called a turn. With Number of turns you define how many strands your coil has.

Fill Factor/Cross section area​

The Fill Factor defines the proportion of the solid inductor area that is occupied by strands.

The Cross-Sectional Area represents the total area occupied by the strands themselves.

Example:

If you define a 10×10 mm square as the Stranded Coil Source (represented by the blue area) with:

• 1 turn, and
• Strand radius of 5 mm,

the fill Factor and Cross-Sectional Area (represented by the green area) can be calculated as follows:

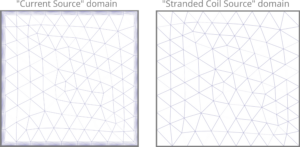
Mesh​

The Stranded Source domain simplifies the geometry and reduces the element count, making simulations more efficient. Unlike the Current Source domain, the Stranded Coil Source domain does not require resolving the skin layer, which can significantly save computational resources.

Important: Ensure that the mesh in the Stranded Coil Source domain is uniform for accurate results.